
 Hover over the painting to magnify (there may be an initial delay while the magnified image is loaded)
Hover over the painting to magnify (there may be an initial delay while the magnified image is loaded)Sir Thomas Monnington (1902-1976):
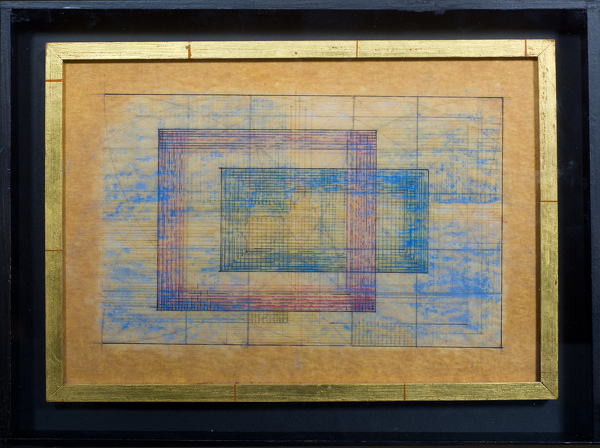
Study 1 for Reciprocity, c. 1970
Framed (ref: 5888)
Chalk, pastel and pen and ink on tracing paper
6 1/2 x 9 1/2 in. (16.5 x 24 cm)
See all works by Sir Thomas Monnington chalk design ink pastel pen and ink Canney Abstract Art
Provenance: The Artist's Estate; Private collection
About Reciprocity:
EXHIBITED: R.A 1971 (77); The Fine Art Society, 1997, (162)
Previewing this work in a newspaper interview (Tate archive, source and date not given) Monnington referred to this as only his second picture in two years.This he explained was on account of being “an extremely slow worker” and painting in “a rather old fashioned mixture of eggs, oil and water, which was used by the early Italian painters.”Asked what the finished painting would look like Monnington replied, “it will consist of a lot of squares”.The link that Monnington clearly made with the early Italian painters,not only through the pursuit of perfect proportion but technique demonstrates the underlying continuity in his work.There was no distinction in his mind between figurative and abstract art.“Surely what matters is not whether a work is abstract or representative, but whether it has merit.If those who visit exhibitions would come without preconceptions, would apply to art the elementary standards they apply in other spheres, they might glimpse new horizons.They might ask themselves: is this work distinguished or is it commonplace? Fresh and original or uninspired, derivative and dull?Is it modest or pretentious?( Interview in The Christian Science Monitor 29.5.67).

When the Tate purchased Monnington’s Square Design (1967) he spoke of his abstract paintings as “direct descendants from my ceiling painting in the Council House, Bristol, which was my first departure from purely representational painting. Since them I have been increasingly interested in the subdivisions of surface areas contained in equilateral rectangels (squares) and rectangles derived from square roots. These two-dimensional mathematical relationships suggest to me dimensions in depth, and provide a discipline which at the present time I find as necessary and interesting as that imposed previously in representational painting... You can cut out the blurb if you wish, but I was trying for my own edification to put into words what I think I have been trying to do in the last ten years”, (letter of 12th June 1968)
 British Abstract Art
British Abstract Art SOLD
SOLD




